Abstract

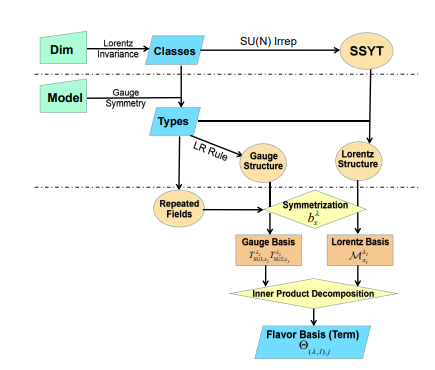
We present a complete list of the dimension 8 operator basis in the standard model effective field theory usinggroup theoretic techniques in a systematic and automated way. We adopt a new form of operators in terms of the irreducible representations of the Lorentz group, and identify the Lorentz structures as states in a SU(N) group. In this way, redundancy from equations of motion is absent and that from integration-by-part is treated using the fact that the independent Lorentz basis forms an invariant subspace of the SU(N) group. We also decompose operators into the ones with definite permutation symmetries among flavor indices to deal with subtlety from repeated fields. For the first time, we provide the explicit form of independent flavor-specified operators in a systematic way. Our algorithm can be easily applied to higher dimensional standard model effective field theory and other effective field theories, making these studies more approachable.

https://arxiv.org/pdf/2005.00008.pdf